Analog Electronics Chapter 4: OPAMP Applications — Adders, Subtractors, Differentiators, and More!

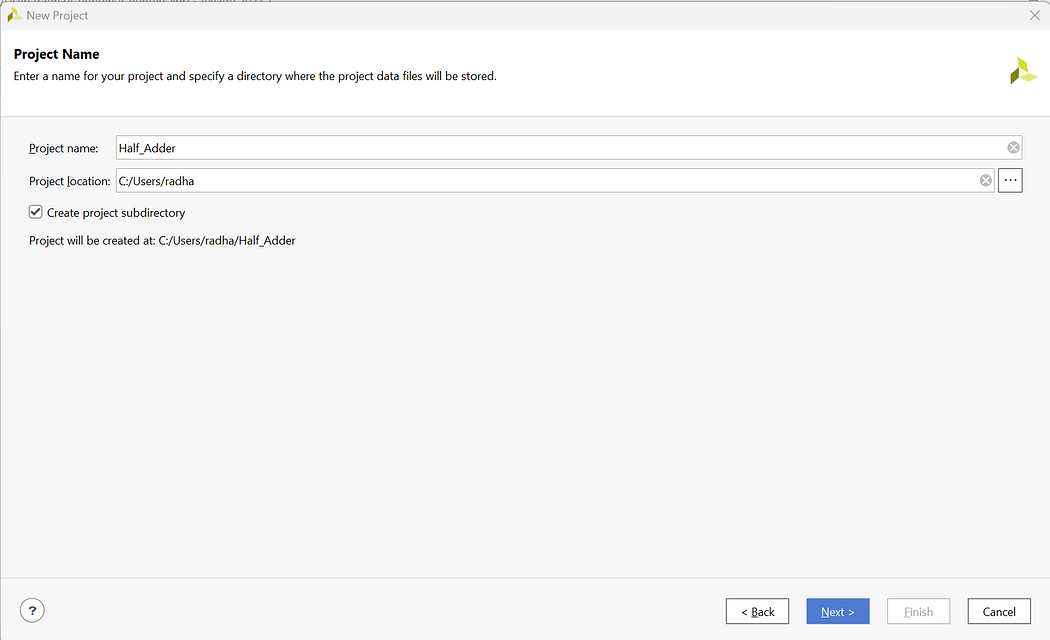
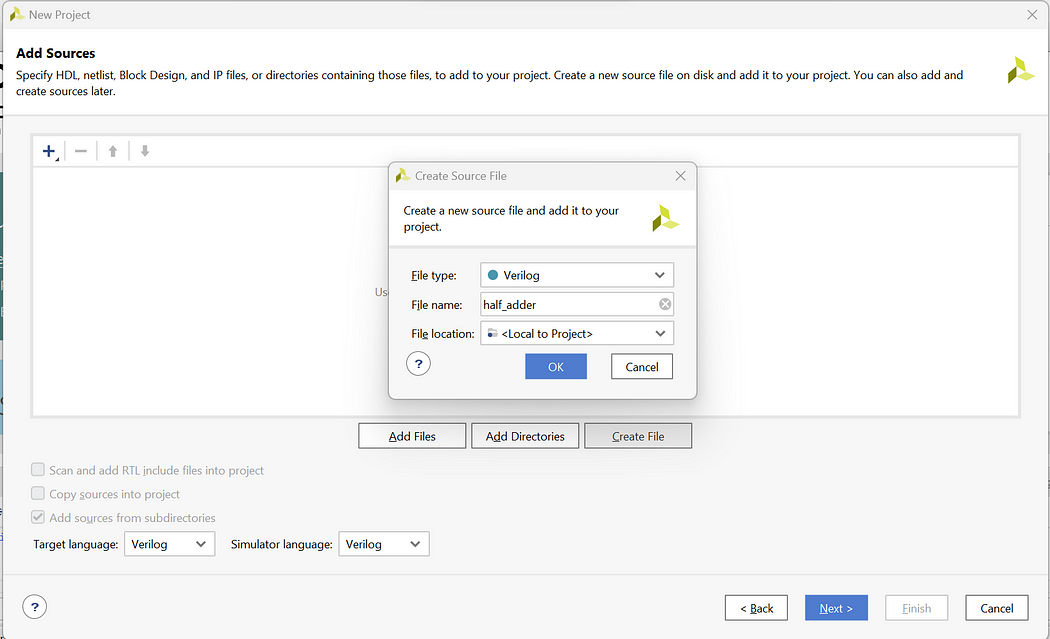
Operational amplifiers (OPAMPs) are incredibly versatile components in analog electronics, offering a wide range of applications. Beyond their basic inverting and non-inverting configurations, OPAMPs can perform complex mathematical operations such as addition, subtraction, differentiation, and more. This chapter will explore these key applications, showing how OPAMPs serve as essential building blocks in signal processing and analog computation.

1] OPAMP as an Adder (Summing Amplifier)

One of the most practical applications of an OPAMP is as an adder (or summing amplifier). This circuit combines multiple input signals and outputs their sum, scaled by the feedback resistor network. The inverting and non-inverting configurations can both be used for this purpose.

Below is the diagram showing the OPAMP as a Summing Amplifier in the inverting configuration:

As shown in above figure, V1, V2, and V3 are three inputs fed to the inverting input through input resistors R1 , R2, and R3. Since the inverting input is at virtual ground, the three currents I1, I2 and I3 are given by:

I1=V1/R1
I2=V2/R2
I3=V3/R3

By Kirchhoff’s Current Law (KCL), the current through the feedback resistor Rf is equal to the sum of these input currents, but it flows in the opposite direction:

If = -Iin
(Vo/Rf) = -[I1+I2+I3]
Vo = -Rf[(V1/R1)+(V2/R2)+(V3/R3)]

This is the general formula for an inverting summing amplifier.

if R1=R2=R3 then
Vo = -(Rf/Rin)[V1+V2+V3]

if (Rf/Rin) = 1 then
Vo =-[V1+V2+V3]

This means the output is simply the inverted sum of the input voltages. In this way, an op-amp configured as an adder effectively combines multiple input signals into a single output, allowing for straightforward manipulation of complex signals. This configuration is essential in various applications, enabling efficient signal processing and integration in electronic circuits.

2] OPAMP as a Subtractor (Differential Amplifier)

An OPAMP can also be configured as a subtractor, allowing for the subtraction of one signal from another. This configuration is commonly used in applications where it’s necessary to compute the difference between two input signals, such as in sensor signal conditioning or instrumentation amplifiers.

The differential amplifier subtracts one input signal from another. The inverting input receives the negative signal, while the non-inverting input receives the positive signal. The output reflects the difference between these two inputs, scaled by the feedback and input resistors.

Below is a diagram showing the OPAMP as a Differential Amplifier:

In this subtractor circuit, V1 is connected to the inverting terminal through resistor R1, and V2 is connected to the non-inverting terminal via another R1. Resistor R2 is placed between the non-inverting terminal and ground, while the feedback resistor R2 connects the output (Vo) to the inverting terminal. The circuit outputs the difference between V1 and V2, scaled by the feedback network, with the inverting terminal at virtual ground for accurate subtraction.

Because of the high input impedance, the current entering the OPAMP is zero.

Therefore, potential at point B is
VB = [R2/(R1+R2)]V2 and Iin = If
(V1-VA)/R1 = (VA-Vo)/R2

As the open loop gain of the OPAMP is very high
From virtual ground concept,
Vo = A(VA-VB) where A → VA = VB

(V1/R1)-[R2/(R1+R2)]x(V2/R1) = [R2/(R1+R2)]x(V2/R2) - (Vo/R2)
(V1/R1)-[R2/(R1+R2)]x(V2/R1) -[R2/(R1+R2)]x(V2/R2) =-(Vo/R2)
(V1/R1)-[V2/(R1+R2)]x[(R2/R1)+1]= -(Vo/R2)
(V1/R1)-[V2/(R1+R2)]x[(R1+R2)/R1]= -(Vo/R2)
(V1/R1)-(V2/R1)= - (Vo/R2)
Vo=-(R2/R1)x(V1-V2)
Vo=(R2/R1)x(V2-V1)
If R1=R2 then
Vo= V2-V1

In this way, an OPAMP configured as a subtractor effectively computes the difference between two input signals, providing a useful tool for applications in signal processing and measurement.

3] OPAMP as a Differentiator

An operational amplifier (op-amp) can be configured as a differentiator, producing an output voltage that is proportional to the rate of change (derivative) of the input signal. This property makes differentiators particularly useful in applications such as waveform shaping, signal analysis, and control systems.

In a basic differentiator circuit, the input signal is applied to a capacitor connected to the inverting input of the op-amp. A feedback resistor is connected from the output to the inverting input. The non-inverting input is typically grounded. This arrangement allows the circuit to respond to rapid changes in the input signal.

Capacitor (C): The capacitor passes changes in voltage but blocks steady-state (DC) signals.
Resistor (R): The feedback resistor determines the output voltage based on the rate of change of the input voltage.

The differentiator circuit is sensitive to high-frequency components due to the nature of the capacitor. As frequency increases, the capacitive reactance decreases, allowing higher rates of change in the input voltage to result in larger output responses.

Derivation:
Here, we know If = -Iin

This states that the feedback current through the resistor R is equal in magnitude and opposite in direction to the input current through the capacitor.

(Vo/R) = -C (dVin/dt)
Vo =-RC (dVin/dt)
When RC =1 then
Vo = =-(dVin/dt)

In this way, an op-amp configured as a differentiator effectively amplifies the rate of change of the input signal, producing an output that is sensitive to high-frequency components. This configuration is vital for various signal processing applications, allowing for the shaping and analysis of waveforms based on their instantaneous rates of change.

4] OPAMP as an Integrator

An op-amp can be configured as an integrator, where the output voltage is proportional to the integral of the input signal over time. This configuration is particularly useful in applications such as analog computation, signal processing, and control systems.

In an integrator circuit, the input signal is applied to the inverting terminal of the op-amp through a resistor, while a capacitor is connected from the output to the inverting terminal. The non-inverting terminal is typically grounded.

Derivation:
Here, we know If = -Iin

If =-(Vin/R)

But if If is capacitive current it is CdVo/dt
Equating the two current expressions gives
CdVo/dt = -(Vin/R)
Rearranging the equation:
dVo = -(Vin dt/RC)

Integrating both sides

∫dVo = -1/RC ∫ Vin dt
Vo = -1/RC ∫ Vin dt
if RC = 1 then

Vo = -∫Vin dt

In this way, an op-amp configured as an integrator effectively converts a time-varying input signal into a corresponding output voltage that represents the accumulated value of the input over time. This configuration is crucial in various applications, facilitating advanced signal processing and control in electronic circuits.

5] OPAMP as Voltage follower (Buffer)

A voltage follower, also known as a buffer amplifier, is a configuration of an op-amp that provides a unity gain (gain of 1) while isolating the input from the output. In this configuration, the output voltage directly follows the input voltage, making it useful for impedance matching and signal buffering.

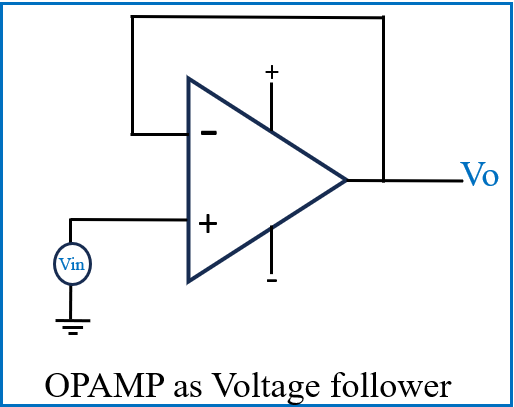
In a voltage follower circuit, the op-amp is connected in a non-inverting configuration. The output is connected directly to the inverting terminal, creating a feedback loop that maintains the output voltage equal to the input voltage.

Here,

Vo = (1+ Rf/Rin)Vin
Vo = (1+ 0) Vin
Vo=Vin
The voltage gain Av is therefore:
Av=Vo/Vin =1

In this way, a voltage follower effectively provides signal isolation and prevents loading effects on the previous stage while maintaining the same voltage level. This makes it an essential component in various applications, such as interfacing between circuits with different impedances.

Conclusion

Operational amplifiers (OPAMPs) are powerful components that play a crucial role in analog electronics. Their ability to perform various mathematical operations — such as addition, subtraction, integration, and differentiation — makes them essential building blocks in signal processing, control systems, and instrumentation. Whether used as summing amplifiers, subtractors, differentiators, integrators, or voltage followers, OPAMPs provide versatility, precision, and reliability. Understanding these fundamental applications allows engineers to design and optimize circuits for a wide range of real-world scenarios, from audio mixers to sensor signal conditioning. Mastering these configurations lays the foundation for more advanced applications in both analog and digital systems.

Understanding the Half Adder: A Fundamental Building Block in Digital Electronics.

In the realm of digital electronics, the ability to perform arithmetic operations is crucial. Among the fundamental components that enable these operations are adders, with the Half Adder being one of the simplest yet essential types. This blog will explore the Half Adder, its components, functionality, and significance in digital circuit design.

What is a Half Adder?

A Half Adder is a digital circuit that performs the addition of two single-bit binary numbers. It produces two outputs: a sum bit and a carry bit. The simplicity of the Half Adder makes it a fundamental building block for more complex arithmetic circuits, such as Full Adders and arithmetic logic units (ALUs).

Theoretical Background

Before delving into the Half Adder, it is essential to understand the basics of binary addition. In binary arithmetic:

1. 0 + 0 = 0
2. 0 + 1 = 1
3. 1 + 0 = 1
4. 1 + 1 = 10 (which is 0 with a carry of 1)

Components of a Half Adder

A Half Adder consists of two primary components:

• XOR Gate: Produces the sum bit.
• AND Gate: Produces the carry bit.

The logical expressions for the outputs are:

• Sum (S) = A XOR B
• Carry © = A AND B

Circuit Diagram

The circuit diagram of a Half Adder is straightforward, with an XOR gate and an AND gate connected as shown below:

Truth Table

The truth table below illustrates how the Half Adder operates for all possible input combinations:

Applications of Half Adder

Half Adders are fundamental components in digital electronics and have several applications, primarily in the construction of more complex arithmetic circuits. Here are some key applications:

1. Building Full Adders: Half Adders are used to construct Full Adders, which can add binary numbers of more than one bit. A Full Adder adds three bits (two significant bits and a carry bit) and produces a sum and a carry bit. By cascading multiple Full Adders (which themselves are built from Half Adders), you can create circuits capable of adding multi-bit binary numbers. This forms the basis of ripple-carry adders and other multi-bit adder architectures.
2. Arithmetic Logic Units (ALUs): Half Adders are integral to the design of ALUs, which perform a variety of arithmetic and logical operations in microprocessors and digital systems. ALUs use Half Adders and Full Adders to perform binary addition, which is a fundamental operation in computing.
3. Digital Signal Processing (DSP): Efficient Data Manipulation: In DSP applications, Half Adders are used for efficient data manipulation and processing tasks that require binary addition. Simple binary calculators use Half Adders to perform basic addition operations. They serve as the foundational units that enable binary addition in these devices.
4. Memory Address Calculation: In memory systems, Half Adders help in the calculation of memory addresses during read and write operations, ensuring data is stored and retrieved correctly.
5. Digital Counters: Half Adders are used in digital counters, where they help in performing the increment operations necessary for counting sequences.
6. Encoders and Decoders: In encoders and decoders, Half Adders assist in converting data between different binary codes, which is essential in various digital communication and storage systems.
7. Error Detection and Correction: Half Adders are used in generating checksums for error detection and correction in data transmission. They help in adding binary values to produce checksums that verify data integrity.

Conclusion

The Half Adder is a fundamental component in digital electronics, serving as a building block for more complex arithmetic circuits. Its simplicity and essential role in binary addition make it a critical topic for anyone studying digital logic design. Understanding the functionality, applications, and implementation of the Half Adder provides a solid foundation for exploring more advanced digital circuits.

For those interested in practical implementations, I have detailed blogs on how to implement a Half Adder using both VHDL and Verilog on Xilinx Vivado. Click on the links below to explore the full implementations and testbench code:

• Implementing Half Adder using VHDL
• Implementing Half Adder using Verilog

Stay tuned for more detailed blogs on combinational circuits and other key topics in digital electronics.

Step-by-step guide on how to design and implement a Half Adder using Testbench code with Xilinx Vivado design tool using Verilog HDL.

Half Adder is a combinational logic circuit that adds two inputs and produces two outputs. The diagram below illustrates the basic block diagram and circuit diagram of a Half Adder, where A and B are the inputs, and Sum and Carry are the outputs.

The table below presents the truth table of a Half Adder.

The output equations of a Half Adder is as follows:

In this project, we will design and implement the Half Adder using Testbench code with the Xilinx Vivado design tool using Verilog HDL.

Step 1: Click on New Project -> Next

Step 2: Enter Project Name and Select appropriate Project Location -> Next

Step 3: Select RTL Project -> Next

Step 4: Click on Create File and create 3 files with file name half_adder-> Next

Step 5: We will not add the Constraint file So click on Next

Step 6: For Default Part click on Next as we are not using any development Board

Step 7: Check the New Project Summary and click on Finish

Step 8: Now you will be prompted with the Define module page but we will create the complete code from scratch so click on cancel and Yes

Step 9: Now on the Home Page click on Sources -> Design Sources -> Non-module Files

Step 10: Enter the below codes in the “half_adder.v” files.

module half_adder(a,b,sum,carry);

input a;
input b;
output sum;
output carry;

xor(sum,a,b);
and(carry,a,b);

endmodule

Step 11: Now to write the testbench code for above design right click on Design Sources -> Add Sources -> Add or create design sources -> Create File -> Add File name as tb_half_adder -> Finish -> Ok -> Yes

Step 12: Now open the testbench file and enter the below testbench code

module tb_half_adder;
reg a;
reg b;
wire sum;
wire carry;

half_adder UUT (.a(a), .b(b), .sum(sum), .carry(carry));

initial begin
$display(“Testing Half Adder gate”);

a = 0; b=0;
#10;
$display(“Input_A = %b, Input_B = %b, Sum = %b, Carry = %b” , a,b,sum,carry);

a = 0; b=1;
#10;
$display(“Input_A = %b, Input_B = %b, Sum = %b, Carry = %b” , a,b,sum,carry);

a = 1; b=0;
#10;
$display(“Input_A = %b, Input_B = %b, Sum = %b, Carry = %b” , a,b,sum,carry);

a = 1; b=1;
#10;
$display(“Input_A = %b, Input_B = %b, Sum = %b, Carry = %b” , a,b,sum,carry);

$finish;
end
endmodule

Here, in testbench code we do not define values in entity hence we have kept it empty. Then in architecture we have copied the components of half_adder and defined signals for connecting the ports of half adder. Inside the process statement we write all 4 test cases from truth table and define the respective delays.

Step 13: Now as we have written all the codes let’s launch the simulation. Enter launch_simulation in the Tcl Console and press Enter.

We have successfully implemented half adder with testbench code. Click on Zoom Fit to see the output waveform more clearly and verify the outputs.

Like, Share, and Follow me if you like my content.
Thank You